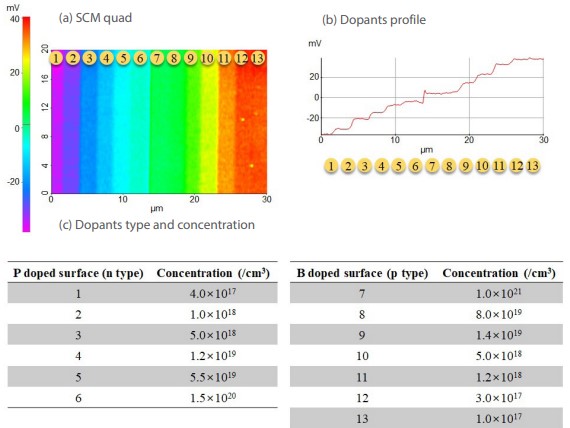
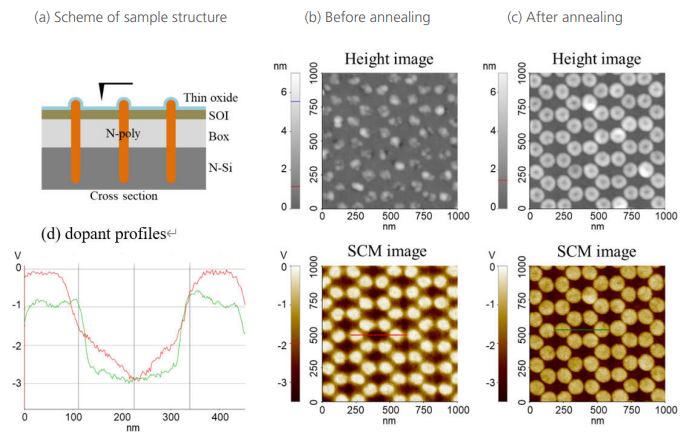
Scanning Capacitance Microscopy (SCM)
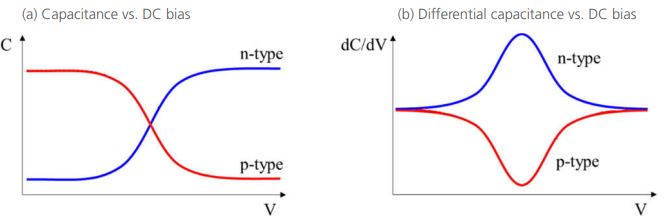
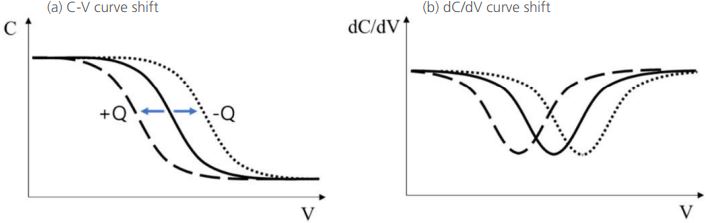
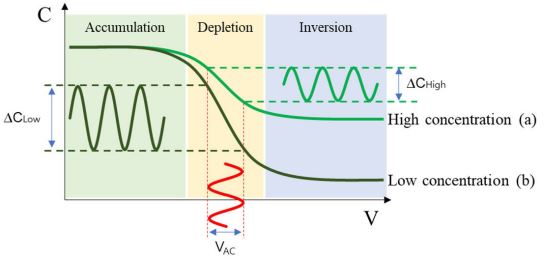
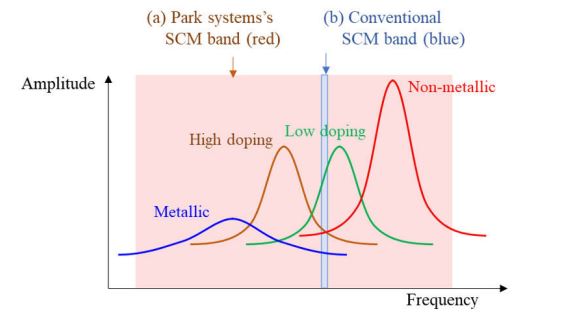
Our SCM mode provides doping concentration information over the sample surface by measuring the capacitance change between tip and sample. the module enables a variable resonator frequency, which allows a wide RF bandwidth capable of monitoring a large range of doping concentrations by selecting the most sensitive frequency of the resonator for a specific doping range.